Statistics Definitions > Point Estimate
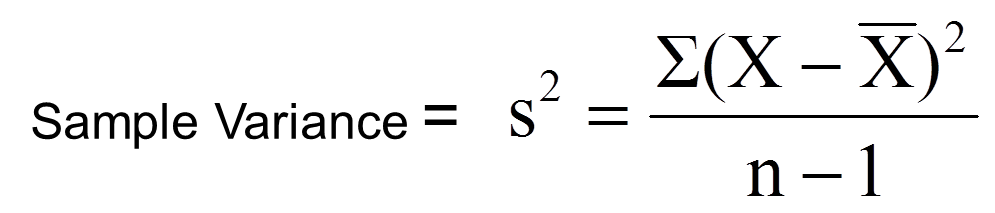
What is a Point Estimate?
Watch the video for an overview and example, or read on below:
A point estimate is a single value that used to estimate an unknown population parameter. For example, if you want to know the average age of pound dogs in the United States, you might use the average age of a sample of pound dogs — taken from a handful of animal care centers — as a point estimate.
In simple terms, a point estimate is a piece of information in an observed sample that is our “best guess” of the information we would find in a population parameter.
In more technical terms, a point estimate arises from applying point estimation to a set of sample data. These estimates are single values. In comparison, interval estimates — such as confidence intervals — contain a range of values.
Point estimate examples
Any statistic can be a point estimate. A statistic is an estimator of some parameter in a population. For example:
- The sample standard deviation (s) is a point estimate of the population standard deviation (σ).
- The sample mean (̄x) is a point estimate of the population mean, μ.
- The sample variance (s2) is a point estimate of the population variance (σ2).
As an example, let’s say you wanted to find an estimate for the mean blood pressure of Olympic athletes. The mean running time of a random sample of Olympic athletes would be a point estimate of the mean blood pressure for all Olympic athletes.
How to find a point estimate
Common methods to find point estimates include:
- Bayes Estimators: In Bayesian statistics, a point estimate is called a Bayes estimator. These minimize the expected value of a loss function, which measures the distance between the estimator and parameter. Common Bayes estimators include the maximum a posteriori (MAP) estimator, posterior mean and posterior median.
- Best Unbiased Estimators (BLUE): Various unbiased estimators can approximate a parameter. In other words, a BLUE estimator is the one closest to the true value of the parameter on average, with the least amount of variability. The “best” one depends on the specific parameter under consideration. For example, when estimating variance, the estimator with the smallest variance is considered the “best”.
- Method of Moments: This BLUE method works by equating the sample moments to the population moments. This method hinges on the law of large numbers and uses relatively straightforward equations to determine point estimates. However, it often lacks precision and can be biased [1]. Maximum likelihood estimation can be more accurate in many cases.
- Maximum Likelihood: This method leverages a model (such as the normal distribution), using the values within the model to maximize a likelihood function. This yields the most probable parameter for the chosen inputs.
Three-point estimates
A three-point estimate is a way of estimating a variable by taking into account three values:
- Optimistic estimate: The best-case scenario — the fastest time to task completion.
- Most likely estimate: The most probable time it will take to complete the task. It is based on the team’s experience and knowledge of the task.
- Pessimistic estimate: The worst-case scenario — the longest time it could take to complete the task.
Three-point estimates are used by a wide variety of people and organizations, including project managers, engineers, and business analysts.
For example, if you’re estimating the time needed to build a fence, your optimistic estimate might be 2 days, your most likely estimate 5 days, and your pessimistic estimate 12 days.
The three-point estimate can be used to find a confidence interval, a range of values that are likely to contain the true parameter value. In this case, the confidence interval would range from 2 to 12 days. If you calculate this interval with a 5% alpha level, that’s a 95% probability that the fence construction time falls within this range.
A three-point estimate provides a more precise estimation than a single point estimate as it acknowledges the built in uncertainty in any estimate. However, it may require more time to calculate compared to a single estimate. In addition, interpreting the results may be more complex.
References
- Topic 13 Method of Moments. 13.1 Introduction