If you take a population sample, you must use a formula to figure out what sample size you need to take. Sometimes you know something about a population, which can help you determine a sample size. For example, it’s well known that IQ scores follow a normal distribution pattern. But what about if you know nothing about your population at all? That’s when you can use Slovin’s formula to figure out what sample size you need to take, which is written as
n = N / (1 + Ne2)
Where:
- n = Number of samples,
- N = Total population and
- e = Error tolerance (level).
Need help with a specific homework question? Check out our tutoring page.
Example question: Use Slovin’s formula to find out what sample of a population of 1,000 people you need to take for a survey on their soda preferences.
Step 1: Figure out what you want your confidence level to be. For example, you might want a confidence level of 95 percent (giving you an alpha level of 0.05), or you might need better accuracy at the 98 percent confidence level (alpha level of 0.02).
Step 2. Plug your data into the formula. In this example, we’ll use a 95 percent confidence level with a population size of 1,000.
- n = N / (1 + N e2) =
- 1,000 / (1 + 1000 * 0.05 2) = 285.714286
Step 3: Round your answer to a whole number (because you can’t sample a fraction of a person or thing!)
- 285.714286 = 286
Like the explanation? Check out the Practically Cheating Statistics Handbook, which has hundreds more step-by-step explanations, just like this one!
What is Slovin’s Formula?
Slovins’s formula is used to calculate an appropriate sample size from a population.
About sampling
Statistics is a way of looking at a population’s behavior by taking a sample. It’s usually impossible to survey every member of a population because of money or time. For example, let’s say you wanted to know how many people in the USA were vegetarians. Think about how long it would take you to call over 300 million people; Assuming they all had phones and could speak!. The problems with surveying entire populations are why researchers survey just a fraction of the population: a sample.
The problem with taking a sample of the population is sample size. Obviously, if you asked just one person in the population if they were vegetarian then their answer wouldn’t be representative of everyone. But would 100 people be sufficient? 1000? Ten thousand? How you figure out a big enough sample size involves applying a formula. While there are many formulas to calculate sample sizes, most of them require you to know something about the population, like the mean. But what if you knew nothing about your population? That’s where Slovin’s formula comes in.
When Slovin’s formula is used
If you have no idea about a population’s behavior, use Slovin’s formula to find the sample size.The formula (sometimes written as Sloven’s formula) was formulated by Slovin in 1960.
The error tolerance, e, can be given to you (for example, in a question). If you’re a researcher you might want to figure out your own error tolerance; Just subtract your confidence level from 1. For example, if you wanted to be 98 percent confident that your data was going to be reflective of the entire population then:
- 1 – 0.98 = 0.02.
- e = 0.02.
Problems with Slovin’s Formula
Slovin’s formula gives you a ballpark figure to work with. However, this non-parametric formula lacks mathematical rigor (Ryan, 2013). For example, there is no way to calculate statistical power (which tells you how likely your study distinguishes an actual effect from one of chance). It’s unclear from any reference texts exactly what the “error tolerance” is (a mean, or perhaps a proportion?).
Some texts call the error tolerance a “tolerance margin of error” (e.g. Ariola, 2006), although it seems to be unrelated to the margin of error used in traditional hypothesis tests. The Margin of Error in that sense is the error associated with a result (for example, you could say 62% of people voted for so and so with a 3% margin of error). From the context, it’s almost certainly another name for the alpha level.
The lack of precision with wording is yet another reason the formula has a poor reputation in academia. But perhaps the biggest reason that the formula isn’t widely accepted is that is seems to have materialized out of nowhere. In fact, no one seems to even know who Slovin is, or even if he existed at all.
Who Invented Slovin’s Formula?
I love a challenge. Out of curiosity I Googled “Who Invented Slovin’s Formula?” today. I remembered waaayyy back when I first learned about Slovin’s formula, it was attributed to “Michael Slovin” but I was looking for a little more information on him. The top search result was Yahoo! Answers with this response as the Best Answer:
I’m sorry, I couldn’t find any information on the net about the origins of Slovin’s Formula or who developed it. Judging by the lack of answers, it looks like not many people of YA know either. Really sorry I couldn’t help. Xxx :)”
Surely it can’t be that hard to figure out where the formula came from…could it? A search for “Slovin’s Formula” just brings up sites (like this one) describing how to use the formula, but not where it came from. Oddly enough, Wikipedia—the site that has a page for everything (Michigan left, anyone?) doesn’t have one for Slovin’s Formula. It doesn’t even have one for “Slovin.” The plot thickens—
A somewhat hilarious Google search for the person who invented “Slovin’s Formula” revealed why you shouldn’t trust everything you read on the web. Several authoritative (*cough*) posts on Ask.com, Wiki Answers and other “Answer” sites gave the following answers to the question “Who invented Slovin’s Formula”:
- Mark Slovin
- Michael Slovin
- Kulkol Slovin
There’s also some chat over at Wikimedia Talk, on the topic of even if there should be a Wikipedia page on Slovin’s formula at all!
“…the formula itself seems clearly notable as you get quite a number of hits under Google books ([1]). Slovin publication of the formula is however dated 1960 not 1843, but it might have known to others earlier.–Kmhkmh (talk) 09:05, 1 April 2013 (UTC)++”
“Slovin’s formula I find no evidence of these formulas that doesn’t seem to trace back to the same handbooks. There is no author in MathSciNet with the name “Slovin”, and the only published article I could find for a person named “Slovin” in 1960 is an unrelated patent.”
This mention of “Sloven’s formula” in the 2003 book “Elementary Statistics: A Modern Approach” by Altares et. al might provide a clue (note the spelling)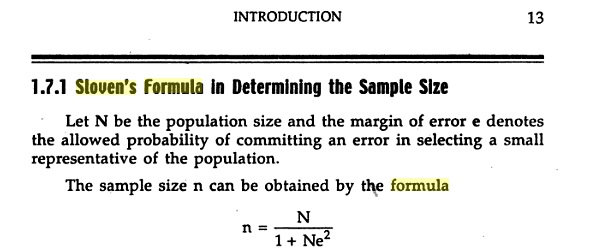 :
:
And Guilford, J.P. and Frucher. B; (1973), Fundamental Statistics in Psychology and Education, New York: MC Graw-Hill does cite Slovin (1960). Now, if I could get my hands on that book, I might be able to solve this mystery!
Taro Yamane’s Formula
Taro Yamane is often credited with an identical formula. However, his formula was published several years after Slovin’s (in 1967).
References
Ryan, T. (2013). Sample Size Determination and Power. John Wiley and Sons.
Yamane, Taro. (1967). Statistics: An Introductory Analysis, 2nd Edition, New York: Harper and Row.