Welch’s Test for Unequal Variances

Hypothesis Testing > Welch’s Test for Unequal Variances

What is Welch’s Test for Unequal Variances?

Welch’s Test for Unequal Variances (also called Welch’s t-test, Welch’s adjusted T or unequal variances t-test) is a modification of Student’s t-test to see if two sample means are significantly different. The modification is to the degrees of freedom used in the test, which tends to increase the test power for samples with unequal variance.

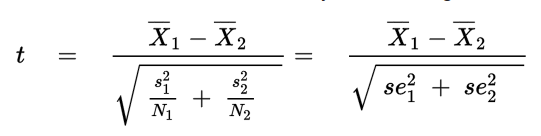
• The null hypothesis for the test is that the means are equal.
• The alternate hypothesis for the test is that means are not equal.

Comparison to Student’s T-Test

Welch’s t-test, unlike Student’s t-test, does not have the assumption of equal variance (however, both tests have the assumption of normality). When two groups have equal sample sizes and variances, Welch’s tends to give the same result as Student’s. However, when sample sizes and variances are unequal, Student’s t-test is quite unreliable; Welch’s tends perform better.

However, it isn’t as simple as choosing Welch’s test when your samples have unequal variances. In fact, some authors (like Zimmerman) caution against testing for variance equality and then choosing the test. Ruxton (2006) states that you should always use Welch’s when comparing central tendency for two unrelated samples.

If you want to run Welch’s—go ahead and run it without testing for variances. It returns the same result as the t-test even if the variances are equal (this however, is not true for small sample sizes, where Welch’s performance is questionable). Most statistical packages have the Welch’s test for unequal variances built in. For example:

• STATA: Run ttest with welch as a parameter (ttest varname₁ == varname₂ [if] [in] , unpaired [unequal welch level(#)].
• SPSS includes Welch’s T as part of the Independent Samples t Test output.
• In Minitab, choose “Basic Statistics”, then “2-Sample t…”. Welch’s is run when the box labeled “Assume equal variances” is left unchecked.

References

Gonick, L. (1993). The Cartoon Guide to Statistics. HarperPerennial.
Everitt, B. S.; Skrondal, A. (2010), The Cambridge Dictionary of Statistics, Cambridge University Press.
Ruxton, G.D., (2006). “The unequal variance t-test is an underused alternative to Student’s t-test and the Mann–Whitney U test.” Behav. Ecol. 17, 688–690. Available here.
Satterthwaite, F. E. (1946). “An approximate distribution of estimates of variance components.” Biometrics Bulletin 2:
Welch, B. L. (1947). “The generalization of ‘student’s’ problem when several different population variances are involved.” Biometrika 34: 28–35.
Vogt, W.P. (2005). Dictionary of Statistics & Methodology: A Nontechnical Guide for the Social Sciences. SAGE.
Zimmerman, D. W. (2004). “A note on preliminary tests of equality of variances”. British Journal of Mathematical and Statistical Psychology. 57: 173–181. doi:10.1348/000711004849222.