The Mellin transform maps a real-valued positive function into a function on the complex plane. It is defined as:
![]()
In general, the integral only exists for complex numbers s = a + jb; a < with a1 < a2, which depend on the function f(t) to transform. As long as you have a reasonably smooth function (a continuous function or piecewise continuous function will also do) that decays rapidly at zero and infinity, the integral converges for any complex number s [1].
While the Fourier transform and Laplace transform were developed to solve physical problems, the Mellin transform was a purely mathematical endeavor. The transform is now used in a variety of problems in engineering and physics, as well as mathematics. These include [2, 3]:
- Analyzing the behavior of functions.
- Estimating integrals.
- Solving Laplace’s equation in polar coordinates.
- Solving a potential problem in a wedge-shaped region, where an unknown function like electrostatic potential or temperature should satisfy Laplace’s equation with certain boundary conditions.
- Solving linear differential equations in electrical engineering.
Mellin Transform: Table of Transforms
The following two tables show a list of common functions (first columns) and their Mellin transforms (second columns):
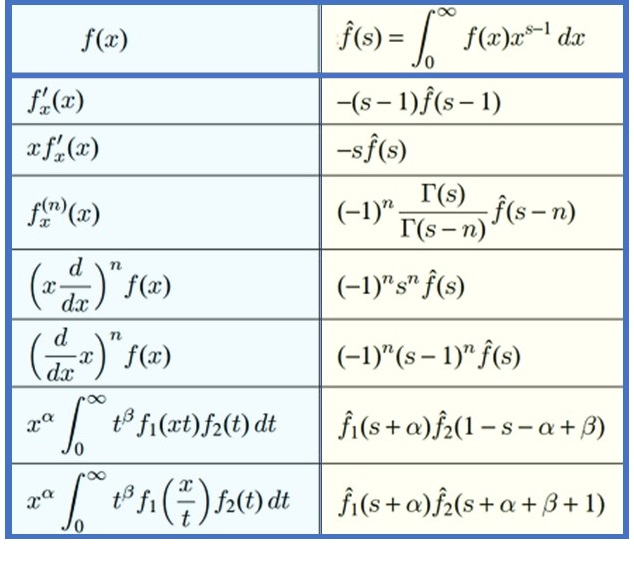
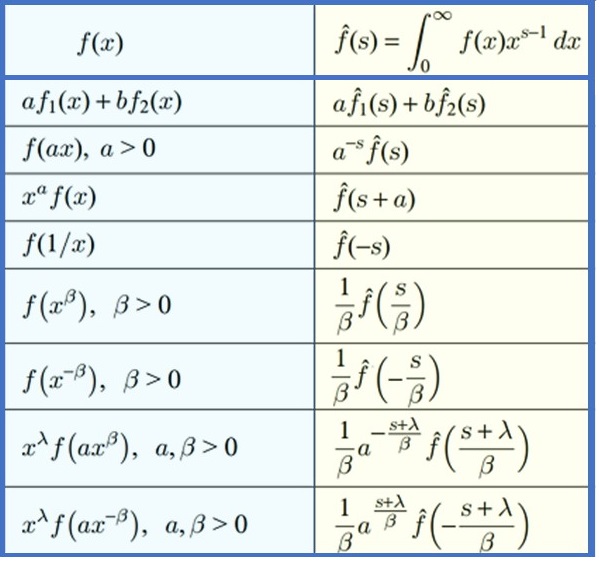
History of the Mellin Transform
The Mellin transform is named after Finnish mathematician R. H. Mellin, who gave the transform and its inverse a systematic formulation. Mellin used the transform to find solutions to hypergeometric differential equations and derive asymptotic expansions. However, Mellin was not the first to discover the transform; it originated with Bernhard Riemann, who used it to study the zeta function.
References
[1] Zagier, D. Appendix. The Mellin Transform and Related Analytic Techniques. Retrieved August 15, 2021 from: https://people.mpim-bonn.mpg.de/zagier/files/tex/MellinTransform/fulltext.pdf
[2] Bertrand, J., Bertrand, P., Ovarlez, J. “The Mellin Transform.” The Transforms and Applications Handbook: Second Edition. Ed. Alexander D. Poularikas. Boca Raton: CRC Press LLC, 2000
[3] Gajjar, J. Mellin Transform. Retrieved August 15, 2021 from: http://oldwww.ma.man.ac.uk/~gajjar/magicalbooks/methods/notest1_2se37.html