What is Infinite Variance?
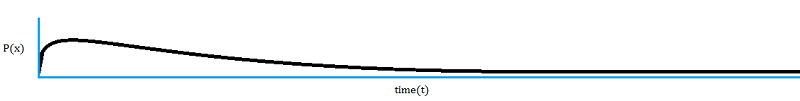
Variance is a measure of how spread out a distribution is. Distributions with infinite variance have fat upper tails that decrease at an extremely slow rate. Intuitively, this means that the distribution will take a very, very long time to disappear; in theory, it never disappears; We say that the upper tail is “unbounded.” The slow decay of probability in this area increases the odds of very extreme values (outliers), “bumps” in the distribution, and other surprising last-minute happenings at some point in the future.
Although the model has infinite variance, this doesn’t imply that the real-world phenomenon being modeled also extends into infinity. It just means that the model is a “good enough” fit to describe the behavior of the phenomenon being studied. Several factors go into the decision to adopt an infinite variance model, including:
- The dataset’s empirical distribution function appears to decline like a power law.
- The empirically measured exponent [for the power law Y = k Xα] is less than 2 (Haring & Lindemann, 2000).
Examples
Models with infinite variance make good models for many hydrological series. Mandelbrot and Wallis (1968) called this the Noah Effect: “By’Noah Effect’ we designate the observation that extreme precipitation can be very extreme indeed,” (p.1). Hurricane Irma—which “…swallowed Jacksonville in record floods”— is an example of this, as was Superstorm Sandy, which caused around $65 billion in damages on the U.S. East Coast.
When a movie studio predicts the profits a movie will make before it’s released, the error is practically infinite. “The average revenue earned by all motion pictures is dominated by a handful of rare blockbuster movies, rare movies so improbable in a Gaussian World that they should never occur (De Vany. 2004).” De The paradox here is that while the infinite variance distribution is a good model for movie profit prediction, a prediction that has an infinite margin of error is no prediction at all.
References
De Vany, A. (2004). Hollywood Economics: How Extreme Uncertainty Shapes the Film Industry. Routledge.
Haring, G. & Lindemann, C. (2000). Performance Evaluation: Origins and Directions, Issue 1769. Springer Science & Business Media.
Mandelbrot, B. & Wallis, J. (1968). Noah, Joseph, and Operational Hydrology. In Water Resources Research.