The equiangular spiral (also called the Bernoulli spiral, logarithmic spiral, logistique, or Spira Mirabilis) is a family of spirals defined as a monotonic curve that cuts all radii vectors at a constant angle [1]. In other words, the spiral forms a constant angle between a line drawn from the origin to any point on the curve and the tangent line at that point [2]. It is this fact — equal angles — that gives the curve its name.
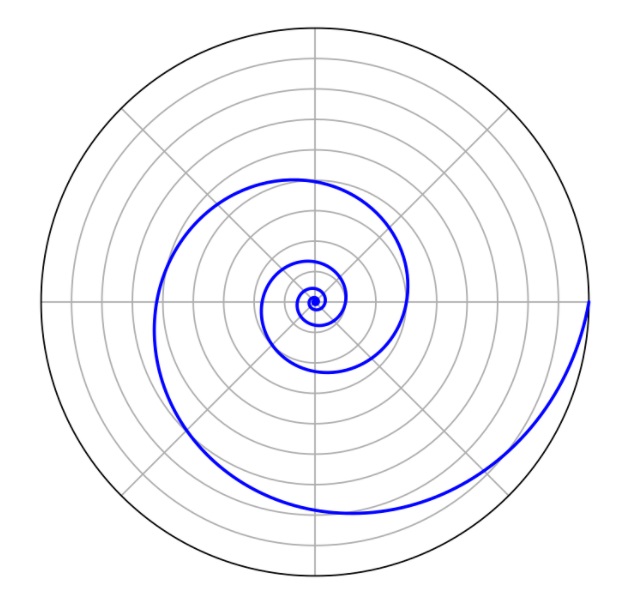
The curve is also sometimes called the geometrical spiral, because a radius’s angle increases in geometrical progression as its polar angle increases in arithmetical progression [3].
Equations for the Equiangular Spiral
The equiangular spiral has polar equation r = r * kθ, where:
- r = initial radius,
- k = a constant > 1 or < 1,
- θ = the angle.
The parametric equation is [1]:
- x = e (t * cot(α)) * cos(t)
- y = e (t * cot(α)) * sin(t)
The Cartesian equation is:
x2 + y2 = e(θ*cot(α)).
Various natural phenomena have the shape of an equiangular spiral, including chambered nautilus shells, the Milky Way galaxy, and arrangements of sunflower seeds on the sunflower.
History of the Equiangular Spiral
The first known construction of the equiangular spiral was in Durer’s 1525 book Udterweysung [4]. The formula was discovered by Descartes [1]. It was later studied by Jacques Bernoulli, who dubbed the spiral spira mirabilis, “the wonderful spiral.” Bernoulli was so enamored with the spiral that he has it engraved on his tomb with the phrase “Eadem mutata resurgo” (Though changed, I rise again the same.).
References
[1] Tully, D. Equiangular Spiral, Logarithmic Spiral, Bernoulli Spiral. Retrieved February 23, 2022 from: https://mse.redwoods.edu/darnold/math50c/CalcProj/Fall98/DarrenT/EquiangularSpiral.html
[2] Spiral. Retrieved February 23, 2022 from: https://mse.redwoods.edu/darnold/math50c/CalcProj/Sp98/GabeP/Spiral.htm
[3] Erbas, A. MATH 7200-Foundations of Geometry. Retrieved February 23, 2022 from: http://jwilson.coe.uga.edu/EMT668/EMAT6680.F99/Erbas/KURSATgeometrypro/golden%20spiral/logspiral-history.html
[4] Albrecht Dürer (1525). Underweysung der Messung, mit dem Zirckel und Richtscheyt, in Linien, Ebenen unnd gantzen corporen