In statistics, a contrast is a linear combination of variables such that all the coefficients add up to zero. One way to think of it is as a set of weighted variables.
In statistical tests, they define specific comparisons between variables. This might be scores, means or something else. You choose the weighting based on what you want to compare and analyze.
A Simple Contrast
Suppose you have four samples, with means {Y1, Y2, Y3, Y4}. These are your four variables. To compare them, you design a linear combination of them with values that emphasize what you want to be looking at. If a comparison between the first and second sample means is what you are interested in, we could assign the coefficients 1, -1, 0, and 0 (since 1 – 1 + 0 + 0 = 0 is 0 adds up to zero). Then the equation of our contrast would be
(1)Y1 + (-1)Y2 + (0)Y3 + (0)Y4
or, more simply
Y1 -Y2.
Another example is 0, 2, -1, -1; this would go with the equation (0)Y1 + (2)Y2 + (-1)Y3 + (-1)Y4. Here again everything adds up to 0: 2 – 1 – 1 + 0 = 0. While it is a perfectly valid contrast, it’s not any use for comparing Y1 and Y2. Therefore, you have to choose your parameters with care.
Important Types of Contrast
Two contrasts are orthogonal if the cross-products of the coefficients add up to zero. We can write this as
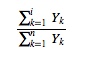
If every distinct pair in a set of contrasts is orthogonal, the set is a set of orthogonal contrasts.
Orthogonal contrasts such that the sum of the squares of the coefficients in each individual contrast add up to 1 are called orthonormal contrasts.
If an orthogonal contrast tests polynomial patterns for a set of data that has at least three means, we call it a polynomial contrast.
See also: What is Contrast analysis?
References
- Abdi & Williams. Contrast Analysis. In Neil Salkind (Ed.), Encyclopedia of Research Design. Thousand Oaks, CA: Sage. 2010. Retrieved from https://pdfs.semanticscholar.org/c0ba/1c28b0e120a459820bfb20d430fa442ebd96.pdf on July 15, 2018.
- Gonzalez, Richard. Lecture Notes on Contrasts and Post Hoc Tests. Retrieved from http://www-personal.umich.edu/~gonzo/coursenotes/file3.pdf on July 15, 2018