The fourth derivative (also called a jounce or snap) is when you take the derivative of a function four times. In other words:
- Find the first derivative, then
- Find the second derivative, then
- Find the third derivative, then
- Find the fourth.
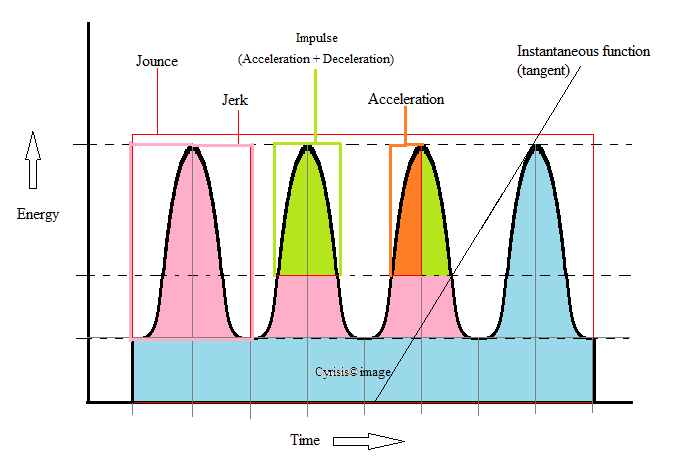
While you can technically take the fourth derivative of any differentiable function, you’ll mostly come across a jounce in physics, which is the fourth derivative of the position function. It tells us the rate of change of the jerk (3rd derivative) with respect to time.
Notation
The fourth derivative is defined by the following equivalent notations:
- y′′′′
- (f′′′(x))′)
- f(4)(x)
Adding primes (′) starts to look a little messy, so most people prefer to use the shortened (f(4)(x)) notation. Be careful to place parentheses around the exponent, because f4 (without parentheses) is exponentiation (i.e. 24 = 16), not differentiation.
Fourth derivative example
Find the fourth derivative of the function:
f(x) = x4 – 5x2 + 12x – 3
Solve using the power rule four times to differentiate exponents.
- f′(x) = 4x3 – 10x + 12
- f′′(x) = 12x2 – 10
- f′′′(x) = 24x
- f(4)(x) = 24
In theory it is possible to continue taking derivatives up to infinity. However, with many equations it will only be possible to go up to the fourth, fifth or possibly the sixth derivatives. If I had continued to the fifth derivative in the example above I would have arrived at zero. Continuing to the sixth and successive derivatives would have also resulted in zero.
Uses for the Fourth Derivative
The fourth derivative is useful in understanding velocity and acceleration. Velocity is the rate at which an object is traveling plus its direction, and acceleration is the rate of change in velocity. The fourth derivative (jounce) tells us the rate of change in the “jerk” part of acceleration— those moments when the acceleration suddenly speeds up (or slows down) such as a lift or elevator ascending (or descending) quickly.
Velocity starts at zero and increases from there. In other words, velocity doesn’t suddenly switch on—it’s the result of acceleration. Likewise, acceleration also grows from zero and doesn’t appear out of nowhere. Therefore, in order for there to be a change in the rate of acceleration there must be jounce. Without calculating the fourth derivative we would be unable to fully understand this process (nor would it be possible for engineers to calculate the safe speeds and elevations at which to operate your favorite amusement park rides).