Contents:
Isotropic Definition
In general, isotropic means uniform in all directions. For example, radiation is uniform in intensity, no matter which direction the measurement is taken in. The opposite is anisotropic, which can be taken as meaning “not uniform.”
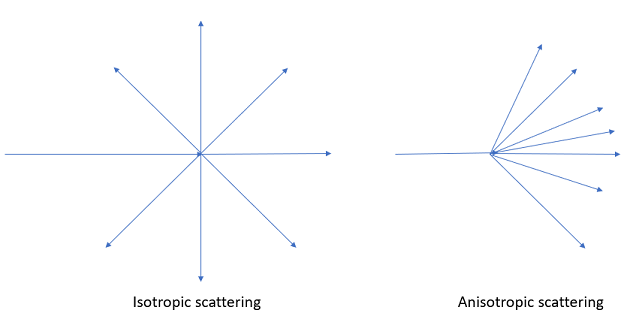
The term has many different meanings in calculus and mathematics. In many cases, they are usually a slight variation on the general definition of isotropy. For example, manifolds with isotropy have the same geometry, regardless of direction. Probability distributions can be isotropic as well, although they are usually defined a little more rigorously than just saying they are “uniform in all directions”. For example, a uniform probability distribution on K is in an isotropic position with a mean of zero and an identity of the covariance matrix.
More Specific Definitions
Some terms require further definition:
1. Isotropic Calculus
A branch of calculus dealing with isotropic pseudo-differential operators, a subclass of pseudo-differential operators with particular decay behavior (the decay is simultaneously in x and the universal set ξ). Defined by symbols a—functions on R2n (Epstein, 2002).
2. Coordinates with Isotropy
A set of coordinates where the physical distance is spatially isotropic. For example, given an infinitesimal change in coordinates a, the distance between (x, y, z) and (x + a, y, z) = the distance between (x, y, z) and (x, y + a, z) = the distance between (x, y, z) and (x, y, z + a).
3. Isotropic Group
Groups that have proper parabolic subgroups defined over k, where a “parabolic subgroup” is defined as one containing a Borel subgroup (Tits, 1964).
4. Isotropic Operators
In image processing, this operator detects edges (i.e. sharp changes in the brightness function) in any direction (Bedros, 2017).
5. Isotropic Position and Distribution
A type of “symmetric” position. Every convex body can come to an isotropic position via an affine map (Fresen, 2011). Probability distributions can also exhibit isotropy.
6. Isotropic Quadratic form
A quadratic form q, defined over a field F, which has a non-zero vector on which the form evaluates to zero (Tsit, 2005).
7. Isotropic Line and Vector
In complex analysis, a line that passes though the origin towards an isotropic vector: A non-zero vector, orthogonal to itself.
Anisotropic Definition
Anisotropic basically means “depends on direction.” It can refer to properties of a wide range of physical and theoretical objects, which have different properties for different directions. Therefore, the exact meaning depends on what specific topic you’re studying. The opposite is isotropic, which doesn’t depend on direction—In other words, isotropy means “uniform in every direction”.
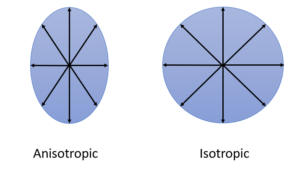
Anisotropic in Calculus
Many terms in calculus can be classified as anisotropic or isotropic, including systems, differential operators and density functions. These definitions often have subtle meanings. For example:
- An anisotropic density function depends on tangent direction (Harrison, 2016).
- Anisotropic tensor calculus is a specific branch of calculus that deals with retaining the same directional class when working with tensors (Javaloyes, 2016).
Meaning in Science
Anisotropy has several different practical meanings in various branches of science. But they all share in common the basic property of not being equal in all directions. For example, growth anisotropy in biology means that growth rates aren’t equal in every direction.
Anisotropic materials have different values for different directions. Natural materials like wood are stronger in one direction (along the grain) and weaker in another (against the grain). If the wood is straight grained, it’s orthotropic, a subset of anisotropy defined as being symmetrical about two or three perpendicular planes. Man-made materials like helicopter blades, reinforced concrete beams and skis are elastically anisotropic (Cowin, 2013).
References
The Baskin Lab. Growth Anisotropy. Retrieved February 6, 2020 from: http://www.bio.umass.edu/biology/baskin/research_anisotropy.htm
Bedros, (2017). Lecture 7: Image Detection. Retrieved January 15, 2019 from: http://www.me.umn.edu/courses/me5286/vision/VisionNotes/2017/ME5286-Lecture7-2017-EdgeDetection2.pdf
Braga de Costa Campos, L. (2014). Generalized Calculus with Applications to Matter and Forces. CRC Press.
Cowin, S. (2013). Continuum Mechanics of Anisotropic Materials. Springer Science & Business Media.
Epstein, C. (2002). Lectures on Indices and Relative Indices on Contact and CR-Manifolds.
Fresen, D. (2011). Comments on the floating body and the hyperplane conjecture.
Graustein, W. C. Introduction to Higher Geometry. New York: Macmillan, p. 121, 1930.
Harrison, J. (2016). General Methods of Elliptic Minimization.
Javaloyes, M. (2016). Tensor Calculus. Retrieved February 6, 2020 from: https://arxiv.org/abs/1602.05492Tits, J. (1964). Algebraic and Abstract Simple Groups. Annals of Mathematics Second Series, Vol. 80, No. 2 (Sep., 1964), pp. 313-329
Tsit, Y. (2005). Introduction to Quadratic Forms over Fields, Graduate Studies in Mathematics 67, American Mathematical Society.