The asymptotic confidence interval is the “textbook” method for constructing confidence intervals. It’s valid for large sample sizes (hence the alternate name, “large-sample” confidence interval) but there are no set guidelines for how large is “large enough” [1]. If you know the distribution for your statistic, you can construct an exact confidence interval. In most cases though, you don’t know the exact distribution, so have to create an approximate confidence interval — an asymptotic one.
Exact vs. Asymptotic Confidence Interval
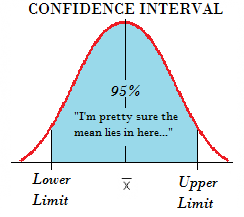
When a statistic’s exact distribution is known, the coverage probability — the probability that the random interval includes the true population parameter — is equal to the confidence level and the confidence interval is exact. In other words, an exact confidence interval will give the exact stated confidence level (e.g., 95%); It is guaranteed that if you were to repeat an experiment or survey over and over again, 95% of the time you would get the same results.
On the other hand, an asymptotic confidence interval will only give an approximation. For example, instead of 95%, you might actually get 91%. One way to deal with this is to use Wilson confidence intervals, which have better coverage rates for small samples.
There is no “right way” to construct an asymptotic confidence interval. However, the larger your sample, the better. Methods for finding asymptotic confidence intervals include maximum likelihood estimators, Z transformation and the studentization procedure [2].
References
[1] Geyer, C. (2003). Stat 5102 Notes: More on Confidence Intervals. Retrieved April 4, 2022 from: https://www.stat.umn.edu/geyer/old03/5102/notes/ci.pdf
[2] Deshmukh, S. & Kulkarni, M. (2021). Asymptotic Statistical Inference: A Basic Course Using R. Springer Singapore.