What is Epsilon Squared?
In statistics, epsilon squared is a measure of effect size (Kelly, 1935). It is one of the least common measures of effect sizes: omega squared and eta squared are used more frequently.
Epsilon Squared Formula
The formula is basically the same as that for omega squared, except that there is one less term in the denominator:
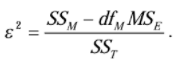
The first SS is the between sum of squares, df is the between degrees of freedom and MS is mean within sum of squares. The last SS is total sum of squares.
These values are obtained from ANOVA output, although epsilon squared itself will usually be calculated by hand (need help with the formula? Check out our tutoring page!). The result will differ very little from omega squared (Carroll and Nordholm, 1975) but will always be slightly larger (Okada, 2013).
Relationship to R2
The Dictionary of Statistics & Methodology (Vogt, 2005) defines epsilon squared as “another name for adjusted R2“. Frieman et. al state something similar: “Epsilon squared (ε2) is the adjusted R2 reported in many data analysis programs.” A glance at the two formulas for adjusted R2 and ε2 may cause you to think they are completely different, when in fact they are equivalent—at least, in SPSS. Adjusted r-squared is the term used for regression analysis calculations, while the equivalent formula is called epsilon-squared in ANOVA (Allen, 2017, p. 382).
Alternative Definition
In computer/hacker jargon, the term epsilon-squared means something else entirely. According to The Jargon File, it is an “insignificant amount”. For example, a single bolt compared to the cost of an entire space rocket is epsilon-squared.
References
Allen, R. (2017). Statistics and Experimental Design for Psychologists: A Model Comparison Approach. World Scientific Publishing Company.
Carroll and Nordholm, (1975). Sampling characteristics of Kelley’s ε2 and Hays w$2. Educational and Psychological Measurement. 35, 541-554.
Frieman et. al. (2017). Principles & Methods of Statistical Analysis.
Kelley, T. (1935) “An unbiased correlation ratio measure.” Proceedings of the National Academy of Sciences. 21(9). 554-559.
Mellinger, C. & Hanson, T. (2016). Quantitative Research Methods in Translation and Interpreting Studies. Taylor and Francis. Retrieved March 9, 2018 from: https://books.google.com/books?id=9TMlDwAAQBAJ
Okada, K. (2013). Is Omega Squared less biased? A comparison of three major effect size indices in one way ANOVA. Behaviormetrika. 40(2). 129-147.
Vogt, W.P. (2005). Dictionary of Statistics & Methodology: A Nontechnical Guide for the Social Sciences. SAGE.