The “epicycles of Ptolemy” refers to a simple model of the universe based on circular motion.
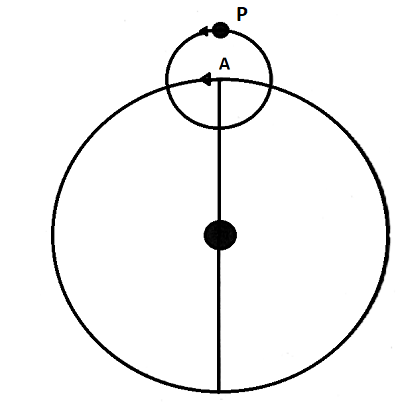
In its simplest form, an epicycle is the path a point in the center of a circle traces as it rolls on another circle. Basically, it’s a small wheel that orbits on a larger wheel. More complicated epicycles can be generated by having a third circle roll on the second, a fourth on the third, and so on. Some epicycles are closed curves (which are also algebraic curves of the form p(x, y) = 0 for a polynomial p) [1]. Rolling circles with irrational radii (irrational means you can’t write it as a fraction) cannot be described by polynomials and therefore are not algebraic.
History of The Epicycles of Ptolemy
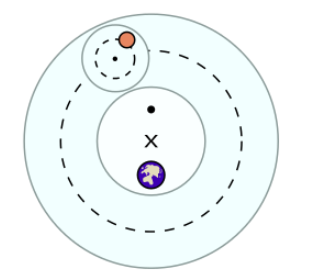
The curves originated with the ancient Greeks, who used them to try and model planetary movement, relative to fixed stars and with a geometry based on the circle. This is, perhaps surprisingly, quite possible! Lagrange [2] showed that motion along a celestial equator can be approximated by epicylic motion; a more modern result can be found in Sternberg [2]. Claudius Ptolemy’s famous astronomical work, the Almagest (“the greatest”). Ptolemy attributed his work to Apollonius (“the great geometer”), who almost certainly mastered conic sections. This is ironic, states J. Stillwell in his book Mathematics and Its History [1], because “epicycles were his candidates for the planetary orbits, destined to be defeated by those very same conic sections.”
Ptolemy made the mistake of considering the rather complicated nature of planetary motion relative to other planets, rather than their more simple motion relative to the sun—which allows for elliptical orbits instead of circles. His attempt at preserving the geocentric model violated the scientific principle of searching for simplicity. He also based his work on three “known” truths of the time [4]:
- All heavenly motion is uniformly circular,
- Heavenly objects do not change (e.g., stars cannot be born or die).
- The universe revolves around the Earth.
References
[1] Stillwell, J. Mathematics and Its History. 3rd Edition. Springer.
[2] Lagrange, J. L. (1772). Recherches sur la maniere de former des tables des
planetes d’apres les seules observations. Mem. Acad. Roy. Sci. Paris. In his
OEuvres 6: 507–627.
[3] Sternberg, S. (1969). Celestial Mechanics. Part I. New York-Amsterdam: W. A.
Benjamin, Inc. Mathematics Lecture Note Series: XXII.
[4] Ptolemy to the Rescue? Retrieved January 22, 2022 from: http://homework.uoregon.edu/pub/emj/121/lectures/ptolemy.html