Composite reliability (sometimes called construct reliability) is a measure of internal consistency in scale items, much like Cronbach’s alpha (Netemeyer, 2003).
It can be thought of as being equal to the total amount of true score variance relative to the total scale score variance (Brunner & Süß, 2005). Alternatively, it’s an “indicator of the shared variance among the observed variables used as an indicator of a latent construct” (Fornell & Larcker, 1981).
Formula
Confirmatory Factor Analysis is one way to measure composite reliability, and it is widely available in many different statistical software packages. By hand, the calculations are a little cumbersome. The formula (Netemeyer, 2003) is:
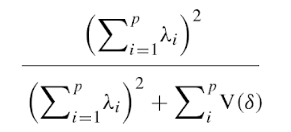
Where:
- λi = completely standardized loading for the ith indicator,
- V(δi) = variance of the error term for the ith indicator,
- p = number of indicators
Thresholds for Composite Reliability
Thresholds for composite reliability are up for debate (a reasonable threshold can be anywhere from .60 and up), with different authors offering different threshold suggestions. A lot depends upon how many items you have in your scale. Smaller numbers of scale items tend to result in lower reliability levels, while larger numbers of scale items tend to have higher levels. That said, Richard Netemeyer and colleagues state in Scaling Procedures: Issues and Applications that it’s “reasonable” for a narrowly defined construct with five to eight items to meet a minimum threshold of .80.
References
Brunner, M. & Süß, H. (2005). Analyzing the Reliability of Multidimensional Measures: An Example from Intelligence Research. Retrieved May 16, 2019 from: http://citeseerx.ist.psu.edu/viewdoc/download?doi=10.1.1.856.4612&rep=rep1&type=pdf
Fornell, C. & Larcker, D. (1981). Evaluating Structural Equation Models with Unobservable Variables and Measurement Error. Journal of Marketing Research Vol. 18, No. 1 (Feb), pp. 39-50.
Ketchen, D. & Berg, D. (2006). Research Methodology in Strategy and Management. Emerald Group Publishing.
Netemeyer, R. et. al, (2003). Scaling Procedures: Issues and Applications. SAGE.