Contents:
- What is a Randomized Clinical Trial?
- Cluster randomization
- Statistics used and their meanings (e.g. CI, hazard ratio, NNT)
- Related Articles
What is a Randomized Clinical Trial?
A randomized clinical trial is an experiment where every person in a trial is randomly assigned to either a treatment group or a control group. A clinical trial is performed in a controlled setting (e.g. a clinic or hospital), targets a specific disease, and has an event as a measure of trial outcome (e.g. cure/no cure).
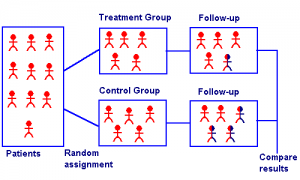
A randomized clinical trial has four elements:
- A treatment group and a control group. The treatment group receives an experimental treatment. The control group might be given no treatment at all, a placebo, or “treatment-as-usual.” The idea is that the control group gives the clinician something to compare the experimental results to.
- Randomization to allocate patients to one of the two groups. This randomization is normally performed by a computer.
- Blinding to prevent the patient or researcher from knowing what group they are assigned to. In a blind trial, the participants don’t know what treatment they are receiving (which may be a placebo). In a double blind trial, neither the participant nor the researcher knows which group the participant is in. Trials are often double-blinded to avoid selection bias on the part of the researcher.
- An ethical reason for the trial.
If a patient decides to participate in a clinical trial, they may not receive any treatment at all if they are assigned to a control group. This is usually not the case for serious diseases like cancer; A control group receives the customary/standard treatment (treatment-as-usual). However, if no standard treatment is available, the control group would receive a placebo.
Randomized Clinical Trial: History
The first fully controlled clinical trial was conducted by J.B. Amberson, B.T. McMahon and Pinner in 1931. Twenty-four tuberculosis patients were either given treatment (a sodium-gold concoction called sanocrysin) or were given no treatment at all. A coin flip decided who received treatment and who did not; The control group received injections of distilled water. The findings were that the two groups had no significant difference in recovery, so sanocrysin was discredited as a treatment for TB. Statistician Sir Austin Bradford Hill introduced the idea of random numbers to assign patients in the 1940s—a process that is still used today.
Phases of Randomized Clinical Trial
- Phase 0 Clinical Trial.
- Phase 1 Clinical Trial.
- Phase 2 Clinical Trial
- Phase 3 Clinical Trial
- Phase 4 Clinical Trial
What is a Phase 0 Clinical Trial?
 A phase 0 randomized clinical trial is the first time a drug is tested on people. The trials follow the FDA’s Investigational New Drug (IND) guidelines. The goal is to find drugs that are suitable for full-blown clinical testing. Usually, these trials have:
A phase 0 randomized clinical trial is the first time a drug is tested on people. The trials follow the FDA’s Investigational New Drug (IND) guidelines. The goal is to find drugs that are suitable for full-blown clinical testing. Usually, these trials have:
- Small numbers of patients: 6 to 15 is typical.
- Short time span — less than two weeks.
- Small doses of drugs. These can be microdoses all the way up to the No Observed Adverse Effect Level (NOAEL). The NOAEL is the highest dose where adverse or toxic effects were not seen.
- No direct benefit to the patient.
Phase 0 trials do not test a drug for safety (tested in Phase 1) or effectiveness (tested in Phase 2).
Purpose
The purpose of a phase 0 clinical trial is to gather information about a potential drug. Researchers want to know how a drug works in the body and if it reacts in the body as expected.
Although phase 0 trials are not a requirement for FDA approval, they can help speed up the process. Drug companies often use these trials to decide which drug from a variety of candidates is the best for further testing. Phase 0 trials can eliminate drugs from further testing if they don’t behave as hoped for.
As the purpose of the trial isn’t a direct benefit to patients actually in the trial, people usually enroll to help find a cure for future patients. Sometimes enrollees are patients with advanced disease who have no other treatment options.
What to Expect in a Phase 0 Trial
Patients is these small, short-lasting trials are usually volunteers willing to accept the risk of taking a new, untested-on-humans drug. Because very small doses of the drug (typically less than 1%) are used, there is rarely a direct benefit to the patient enrolled in the trial. Small drug doses do carry a lesser risk of side effects, but exactly what side effects might happen are unknown.
In addition to being given a drug that has not been tested on humans before, patients may be required to undertake further tests and procedures, like:
- Medical imaging (e.g. x-rays, MRIs),
- Lab tests and procedures including blood work and biopsies,
- Interviews/Questionnaires.
Preclinical Testing
Before drugs are given to humans, they are first tested on animals. Exactly what testing is required depends on the dose for the phase 0 trial. For example, a single microdose might need 7 days of testing on rodents while larger doses need 14-day repeated-dose tests on rodents and non-rodents. Drugs are also tested on surrogate tissue and/or tissue from human biopsies.
Stats in Phase 0 trials
The main goal of a Phase 0 trial is to streamline the drug approval process. However, these trials can also provide valuable stats for use in future trials, like:
- A threshold for treatment effects on biomarkers, measured with Binomial tests. This can be figured out for individual patients at specific doses with specified false positive rates (e.g. 5%). For example, a threshold value for drug X might be set where the drug performs as expected on a biomarker 75% of the time.
- Reasonable levels of false positives (e.g. 10%).
- Potential drug efficacy thresholds can be figured out for specific doses, for detection with high statistical power.
- Intra-patient variability: Different drug doses given at different times to the same patient might give different responses.
- Primary endpoints: are measures of if drugs work or not (i.e. is there any clinical significance). For example, an endpoint could be the difference in survival time between experimental groups and control groups.
Other Names
Phase 0 clinical trials are also called:
- Exploratory Investigational New Drug (IND) studies.
- First-in-Man trials.
- Pilot studies.
- Pre Phase 1 trials.
What is a Phase 1 Clinical Trial?
 A phase 1 randomized clinical trial tests a new drug for safety and side effects. Goals of this phase are to find out:
A phase 1 randomized clinical trial tests a new drug for safety and side effects. Goals of this phase are to find out:
- How well the drug is tolerated,
- How much drug can be given without severe side effects,
- How much drug is needed and how often,
- What delivery method (e.g. injection into a vein, IV drip, or taken by mouth) works best.
- For vaccines, if the drug creates an immune system response.
The trials can last up to about a year and generally include 10-100 people. Placebos (sugar pills) are not used in a phase 1 clinical trial. In other words, every person in the trial will receive the experimental drug. According to the FDA, 30% of drugs fail this stage and 70% move on to a Phase 2 clinical trial. Patients are usually accepted into a single phase of a clinical trial, so people in phase 1 will probably not be in phase 2 or beyond.
Although it’s often the case that the drug has never before been tested in humans, phase 1 trials can sometimes be preceded by a phase 0 clinical trial where a few people are given a tiny amount of the drug.
Purpose
The goal of a phase 1 clinical trial is to find the maximum safe dose. This is achieved by slowly increasing the dose of the drug and monitoring side effects. Although a phase 1 isn’t designed to test how well a drug works (that’s reserved for Phase 2 clinical trials), doses are given in large enough amounts that a patient might see improvement in their condition.
Risks and Benefits
In a phase 1 trial, participants are divided into groups; The groups receive different amounts of the drugs (e.g. low dose, medium dose, high dose). Patients will generally not know which group they are assigned to. Randomization and blinding may be used to prevent researchers and patients from knowing who is in which group.
Risks include:
- The trial may be stopped early if one group of patients responds better than another group.
- Patients in low-dose groups may receive too little of the drug to be of any benefit.
- Patients may receive too much of the drug — which may cause severe side effects. Because the drugs have typically not been tested on humans before, what side effects may happen can be unknown. Severe side effects can bring an early end to the trial.
Benefits include:
- Most volunteers in phase 1 are paid to be in the trial,
- For some people, clinical trials might be their only hope for finding a cure or symptom relief for a rare or terminal illness.
Issues with Finding Participants
A sample is a small portion of a population. For example, if you have a population of 10,000 people with a certain kind of cancer, a sample might consist of 150 people. Samples in most clinical trials are convenience samples, which means that they are targeted towards a very particular segment of the population (i.e. “all patients at Mercy General with pancreatic cancer”) because that’s the group of people who are readily available. As the samples are not drawn randomly from the entire population (i.e. “all people in the USA with pancreatic cancer”), it’s difficult to run statistical tests on any data. That said, data has to be produced (otherwise no drugs would ever be accepted by the FDA), so researchers use reasonable models (like the binomial distribution for small samples or the normal distribution for larger samples) to create conservative statistics from the research results; Conservative statistics err on the side of caution.
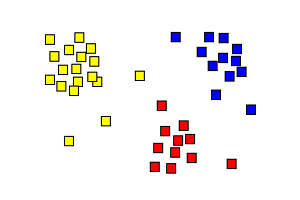
- Geographic location,
- Age,
- Gender.
The groups don’t have to be clusters of people. For example, they could be:
- Community organizations,
- Clinics,
- Countries.
The groups are always pre-existing, rather than being created by the researcher.
Experiments that use cluster randomization are sometimes called cluster randomized trials (CRTs) or group-randomized trials.
When to Use CRTs
CRTs are often used to measure the effectiveness of management strategies and public health interventions. They are sometimes used in a clinical setting to study drug effectiveness (Mazor et. al, 2007). In general, they are a good choice when looking at decisions to treat or not treat entire groups (e.g. whether to immunize groups or provide mosquito netting for an entire geographic area) or when contamination risk is high. Contamination means that individuals are in close contact and influence (“contaminate”) each other. For example, if you want to study how diet affects prison inmates, this carries a high risk of contamination as inmates may swap or share food.
CRTs vs. Individually Randomized Trials
Trials that use cluster randomization are reported and analyzed in the same way as trials that randomize individuals. However, instead of analyzing the trial at the individual level, it’s analyzed at the cluster level. “Cluster level” just means that the results for the entire cluster is looked at, instead of one level further down—the individual level.
Cluster randomized designs usually have lower statistical power than similarly sized experiments that randomize individuals instead of clusters. They also tend to have a higher number of false positives (Type I errors). However, the design has many benefits including better cost efficiency, a lower risk of experimental contamination, and a lower risk of noncompliance from study participants.
References
Donner, A. & Klar, N. (2010). Design and Analysis of Cluster Randomization. Wiley.
Mazor, K. et. al. (2007). Cluster Randomized Trials: Opportunities and Barriers Identified by Leaders of Eight Health Plans. Med Care, 45:S29-S37.
Statistics in Clinical Trials
Some common statistical concepts you’ll likely come across in clinical trials are:
1. Confidence Intervals
Confidence intervals give you a range of values which probably contains the “true” value. For example, let’s say the average time for a cure was 5-9 days with a confidence level of 99%. That means researchers are very confident that if the experiment was to be repeated again, the average cure time would be between 5-9 days.
2. Hazard Ratio
The Hazard Ratio is a measure of whether patients in a treatment group are progressing at different rates than the control group. Any ratio above 1 usually indicates that the experimental group had better results (e.g. healed faster) than the control group. A ratio under one means that the experimental group had worse results than the control group.
3. Mean and Median
The mean and median are measures of “the middle.” They give you an idea of where you can expect to find average results. See:
What is a mean?
What is a median?
4. Number Needed to Treat (NNT)
The Number Needed to Treat is how many people have to be treated in order to prevent one extra adverse outcome; In other words, it’s the number of people who need to be treated in order for one of them to have a positive outcome compared to a control group.
5. Odds Ratio
An odds ratio (OR) is a measure of association one property A and a second property B in a population. For example, let’s say the OR was 2 for a group of people who ate burgers at a potluck and became ill with E-coli . This means that ill people were twice as likely to eat burgers at the potluck as well people.
6. Primary Endpoints
Primary endpoints — measures of if drugs work or not — are often tweaked in a Phase 1 clinical trial. They are the most important measure of trial “success.” For example, an endpoint could be:
- The difference in survival time between experimental groups and control groups.
- Proportion of patients with side effects.
- Average decrease in cell count over a one week period.
7. P-values
The p-value (probability value) is a number between 0.00 and 1.0. It’s used to show the strength of a conclusion in a trial. Small p-values (under about 5%) indicate strong results. The smaller the p-value, the stronger the conclusion.
8. Statistical Significance
A statistically significant result in a trial means that the trial results show significant (promising) findings and that they have been tested with an acceptable statistical test.
What is a Phase 2 Clinical Trial?
 In phase 2 clinical trials, an experimental drug is given to a large number of people to find out how effective the drug is at curing disease or alleviating symptoms.
In phase 2 clinical trials, an experimental drug is given to a large number of people to find out how effective the drug is at curing disease or alleviating symptoms.
Phase 2 Randomized Clinical Trials are the third step in bringing a drug to market. The first phase (phase 0) tests tiny amounts of a drug on a few people and gathers information about how the drug works in the body. The second phase (phase 1) tests the drug for safety by giving increasing doses to small groups of people.
Elements of a Phase 2 Trial
A phase 2 clinical trial:
- Tests a drug’s effectiveness, safety, and dosage,
- Lasts about two years,
- Generally has around 30-120 participants. Randomized phase 2 trials, where different groups of people are given different doses of the drug, might have several hundred participants,
- Target people who actually have the disease the drug is intended to treat (unlike phase 1 trials, which may include healthy volunteers).
- Many include a placebo (“sugar pill”), where a control group is given an inactive substance instead of an experimental drug. If a placebo is unethical (e.g. for cancer patients), a “Usual Treatment” will be given instead of a placebo.
- Is usually double-blinded to prevent patients and researchers from knowing who is receiving which treatment.
If a potential drug is deemed safe and effective after a phase 2 trial, the next step is a Stage 3 Clinical Trial.
Statistics in Stage 2 Trials
For many common statistics terms used in clinical trials, see Statistics in Clinical Trials, which explains confidence intervals, endpoints and more. Some statistics are of particular important in phase 2 trials:
- Primary endpoints: One goal of a stage 2 clinical trial is to further investigate endpoints. For example, does the drug extend life?
- Researchers might also interested in figuring out exact confidence intervals for the trial results. For example, the mean life expectancy might be extended by 7-9 months. This means you can expect (with fairly high confidence) that anyone taking the drug will see an improvement of life expectancy anywhere between 7 and 9 months.
- Two-stage designs are ways to stop ineffective treatments early — saving costs and minimizing risks to patients. For example, Gehan’s two-stage design suggests figuring out the minimum number of people necessary to achieve a single response. For example, if you test 12 people, you might expect one response. Test 12 people, then declare that stage a “success” or “failure”. Move on to large scale testing only if the first stage is a success.
What is a Phase 3 Clinical Trial?

A phase 3 Randomized Clinical Trial is a large scale drug trial to find out if a drug is effective or not for a specific disease or condition. Areas investigated include:
- Safety, including side effects. Although previous tests (Phase 2 trials) have indicated the drug is relatively safe, safety is continually checked.
- Drug dosages: dosage levels will be modified in the trial to find the most effective dose.
- Comparisons to other, usual treatments. Placebos (sugar pills) may be used, except for serious illnesses (like cancer) where that option may be unethical.
If a Phase 3 trial is successful, an application can be made for FDA approval.
Drugs used in a Phase 3 trial have undergone previous testing on animals and small numbers of patients. The trials can last from 2 to 5 years and can involve thousands of participants of different ages, ethnicities, and genders.
Randomization in Phase 3 Trials
Randomization is where patients are randomly assigned to either a treatment group, which receives the experimental drug, or a control group, which receives a placebo or Treatment-as-Usual. Trials may be double-blinded, which means that neither the patient nor the researcher knows which group a patient is assigned to.
Experimental Designs in the Phase 3 Randomized Clinical Trial
Common experimental designs found in phase 3 testing include:
- Crossover design: A crossover design (a type of repeated measures design) is where groups of participants get different-ordered treatments. Group A might get treatment X then treatment Y, while group B gets Y then X.
- Factorial design: Participants typically get two or more treatments at a time. For example, drug A and counseling or drug B and exercise.
- Parallel Design: A parallel design compares two or more treatments. Different groups are given different treatments and then the results are compared. For example, group A might get the experimental drug and group B might get a placebo. It is the “gold standard” for phase 3 clinical trials (1).
Other, less common designs for a Phase 3 Randomized Clinical Trial include:
- Group sequential design: as soon as positive results are found in the trial, the trial is continued. As soon as negative results are found, the trial is ended. As the trial could be stopped or continued at any point in time, the number of patients is not set in advance.
- Historical controls: Patients in the trial get the experimental drug; a control group doesn’t exist in the actual trial — data for control is collected from past events.
- Uncontrolled, single-treatment trials: The experimental drug is given to all participants; there is no control group.
What is a Phase 4 Clinical Trial?
 A Phase 4 Randomized Clinical Trial tests a drug’s effectiveness, safety, and side-effects over the long-term. The trial takes place after the FDA has approved the drug and involves thousands of patients with the indicated disease or condition. This is often the first test of the drug in the “real world.”
A Phase 4 Randomized Clinical Trial tests a drug’s effectiveness, safety, and side-effects over the long-term. The trial takes place after the FDA has approved the drug and involves thousands of patients with the indicated disease or condition. This is often the first test of the drug in the “real world.”
By the time a Phase 4 clinical trial is started, drugs have gone through several stages to reach FDA approval. They have been tested thoroughly enough to ensure they aren’t going to cause calamities when mass-marketed. However, much of the knowledge about a drug’s effectiveness, interactions and side effects is obtained after the drug hits the market.
Design
The Phase 4 Randomized Clinical Trial can be well-controlled, randomized trials. Alternatively, they can be post-marketing surveillance, which means they are observational and non-experimental. Controlled, randomized trials are run in a similar way to Phase 3 trials. The experimental group receives the drug being studied and the control group receives another brand drug or a placebo. Patients are also randomly allocated to a control group or an experimental group. Non-experimental trials mean that the researchers don’t interfere (i.e. they don’t set up an experiment and use a control group) — they only observe and collect data.
Purpose
The primary reason Phase 4 trials are run is to find out if drugs have unexpected or rare side effects. Many Phase 4 studies also study risk factors, test different formulations, dosages, and treatment durations. Interactions with other medications might also be studied, as well as how the drug compares with other medicines on the market.
One important reason that Phase 4 clinical trials exists is to provide information about generalizability. An FDA approved drug has typically been tested on, at most, a few thousand people. Once the drug hits the market, anyone and everyone can take the drug: pregnant women, people with heart conditions, diabetics…the list goes on. It’s only after mass-marketing that the drug is truly studied in depth across a wide range of ethnicities, health conditions and ages.
Randomized Clinical Trial: Related Articles
- Absolute Risk, Relative Risk Calculations.
- What is an Adaptive Design Clinical Trial?
- Adaptive Randomization
- Active Control trials.
- Allocation Concealment.
- Attributable Risk
- What are Binary Endpoints?
- Blinding in Statistics
- What are Composite Endpoints?
- Drug Utilization Studies.
- Effect Modification
- What are Historical Controls?
- Intention to Treat
- Intervention Fidelity
- Large Simple Trial.
- Matching in Statistics.
- Non Interventional Study.
- What is Number Needed to Harm?
- What is Number Needed to Treat (NNT)?
- Pre-Test and Post-Test Probability
- What are Primary Endpoints?
Drug Utilization Studies
 Drug Utilization Studies (DUS) research how medicines are dispensed, prescribed, and used, especially with a view toward identifying problems.
Drug Utilization Studies (DUS) research how medicines are dispensed, prescribed, and used, especially with a view toward identifying problems.
They may answer basic questions such as:
- What medicines people are taking,
- Why they are taking them,
- How much they cost,
- How people budget for them.
Drug utilization studies may also monitor changes in patterns of how various drugs are used. They might also evaluate what happens when there are interventions (for example prohibitions or recommendations) which affect availability.
Drug utilization studies are important for policy makers and all those involved in forming drug policies, and they can give important information to medical professionals and researchers.
Areas Analyzed Through Drug Utilization Studies
Drug utilization studies look at three major areas:
- Medical,
- Social,
- Economical.
In the medical sphere, they look at the benefits of drugs, and how efficient they are in preventing, relieving, and curing diseases. They also look at long and short term risks or adverse affects, and the benefit/risk ratio.
In the social sphere, drug utilization studies examine attitudes toward drugs and health as well as the basis for these attitudes. They look at the improper use of drugs, and drug abuse and dependence. Social injustice issues are also considered, including whether or not important drugs are available to all who need them.
In the economic sphere, drug utilization studies look into the costs of drugs and how that changes between generic or brand name products, or imports vs local products. They also might focus on such things as the way national resources are allocated to drug and health budgets.
Drug Utilization Studies: References
Dukes, M. (1993). Drug utilization studies: Methods and uses. World Health Organization regional publications.
Lee & Bergman. Studies of Drug Utilization. Chapter 24, Pharmacoepidemiology, Fifth Edition. Retrieved from https://onlinelibrary.wiley.com/doi/10.1002/9781119959946.ch24 on April 28, 2017
Mayall & Banerjee. Pharmacovigilance Planning, from Therapeutic Risk Management of Medicines, 2014. Retrieved from https://www.sciencedirect.com/topics/pharmacology-toxicology-and-pharmaceutical-science/drug-utilization on April 28, 2017
Other References for Randomized Clinical Trial:
Amberson, J., McMahon, B. and Pinner, M. (1931). A Clinical Trial of Sanocrysin in Pulmonary Tuberculosis. American Review of Tuberculosis. 24:401-435.
Bacchieri A, Cioppa GD. Fundamentals of Clinical Research: Bridging Medicine, Statistics and Operations, Milano: Springer-Verlag Italia; 2007.
Canadian Cancer Society: http://www.cancer.ca/en/cancer-information/diagnosis-and-treatment/clinical-trials/phases-of-clinical-trials/?region=on
FDA.
Kummar, S. et. al. (2008). Phase 0 clinical trials. Cancer Journal. May-Jun;14(3).
Kupfer, C. (1982). Randomized Clinical Trial. Jpn J Ophthalmol. 982;26(3):241-7.
Crowther, M. (2013). Phase 4 research: what happens when the rubber meets the road? ASH Education Book December 6, 2013 vol. No. 1 15-18.
Spilker, Bert. (1984) Guide to Clinical Trials, Raven Press. Page XXii-Xxiii.
Zhang, D. (2009). Statistical Principles of Clinical Trials. Retrieved February 21, 2017 from: http://www4.stat.ncsu.edu/~dzhang2/st520/520notes.pdf